Sex ratio
in outbreeding populations - Fisher's model of sex- related investment
in inbreeding populations - Hamilton's model
environmentally determined sex ratio
---

---
Fisher's Sex Related Investment Model for Outbreeding Populations
Most populations have a near 50/50 sex ratio -- why?
Proximate answer -- males have half X and half Y chromosomes so that should make a 50/50 sex ratio. But this answer more explains how rather than why?
Some populations have strong deviations from 50/50 even though there is an X/Y system
---
Fisher's Theory of Sex-Related Investment
He noted that in any outbreeding populations half the genes passed on come from males and half come from females
If individuals of one sex is in short supply (and they cost the same to make), then
1) parents who produce children of the rarer sex have a reproductive advantage (because fewer of them contribute half the genes),
2) thus a trait for producing the rare sex is favored by selection because of the higher average reproduction of individuals of that sex.
This kind of selection is called frequency dependent because the advantage of being a male or female producing parent/trait depends on the relative frequency of each type in the population.
When males are scarce it more advantageous to be a male producer, when females are scarce its more advantageous to be a female producer.
Advantages disappear as the population approaches an equilibrium in which neither sex is in short supply.
The result of this kind of selection is to stabilize the population around an equilibrium sex ratio.
---
Why Is Fisher's model called a sex-related investment model instead of a sex ratio model?
This is because it doesn't predict sex ratio per se, but equal population wide investment in maleness and femaleness.
If offspring of each sex cost parents equal to make (investment is the same) then a 50/50 sex ratio is predicted.
But if one sex is more costly for parents to produce, then the predicted equilibrium sex ratio is proportionately biased toward producing more individuals of the less expensive sex.
Ultimately this model connects investment and reproductive success. Because half of reproduction is in one sex then half of total investment (the cost of making offspring) is expected to be committed to that sex.
---
The Evolutionary Stable Strategy
Fisher suggested and ultimate evolutionary answer which was the first of what are now known as an ESS (Evolutionary Stable Strategy model).
Under this kind of model we can set up an initial set of conditions and see what happens to an individual who "plays" a particular strategy.
Under Fisher's SRI model for a out of equilibrium model an individual investing more in the scarcer sex will "win".
---
Building an ESS model
Construct a strategy set -- the array of likely alternative phenotypes that we will test.
Test the performance of the different members of the strategy set to determine which will win (have the highest reproduction).
We can then identify a likely evolutionary outcome.
---
Hamilton's Model of Sex Ratio in Inbreeding Populations
Fisher's model assumes that matings occur in an open market and that ability to gain reproduction by either sex is related to their investment in that sex.
Hamilton considers a different situation in which the opportunity for matings are constrained. The extreme case he considers is one in such a male may breed inside his mother with his sisters before they are born. Under these circumstances how should investment be allocated to the two sexes by the mother?
---
Hamilton's answer is that a Fisherian investment ratio does not give maximum reproduction.
Why?
What would the preferred sex ratio be under these conditions?
---
From the mother's point of view she only needs as many sons as in necessary to fertilize her daughters
Any extra investment in sons is wasted
The investment that might be spent on redundant sons can be more profitably spent on making more daughters
Hamilton predicted that under restricted conditions there should be strongly female biased sex ratios
and investment in tiny (inexpensive) males
---
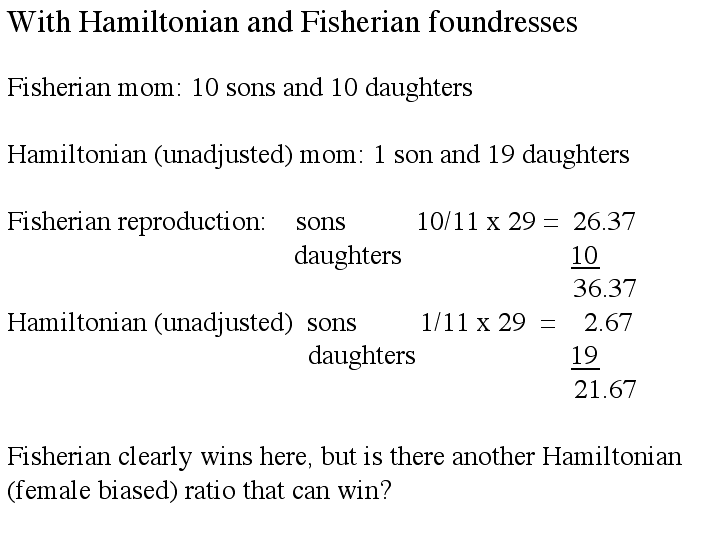
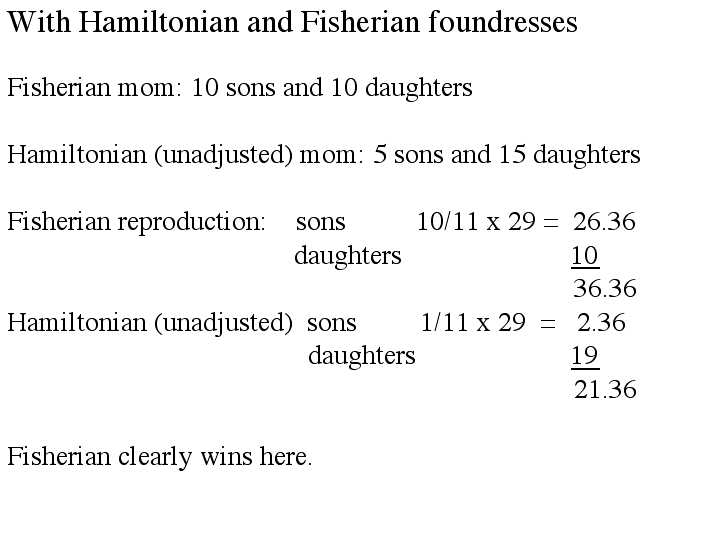
The case of mother's offspring reproducing alone in a host
Consider two mothers that produce 20 offspring, how many grandchildren do they make?
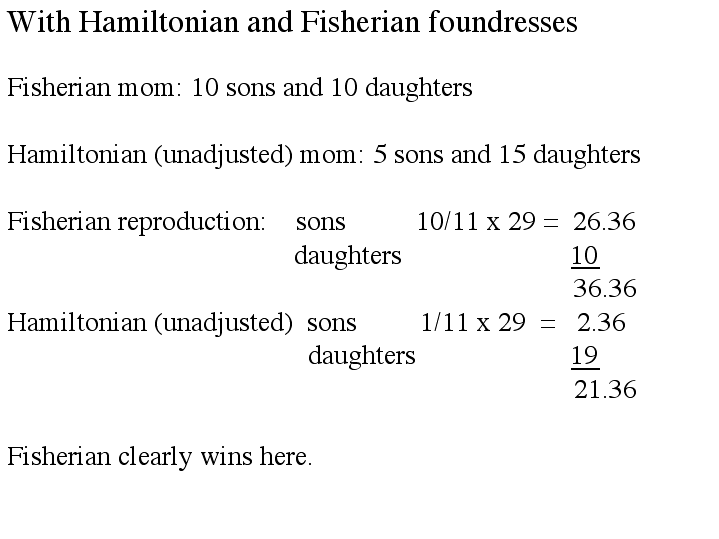
Fisherian mom: 10 sons and 10 daughters
number of grandchildren = 10 x 20 = 200
Hamiltonian mom: 1 son and 19 daughters
number of grandchildren = 19 x 20 = 380
Hamiltonian mom wins
---
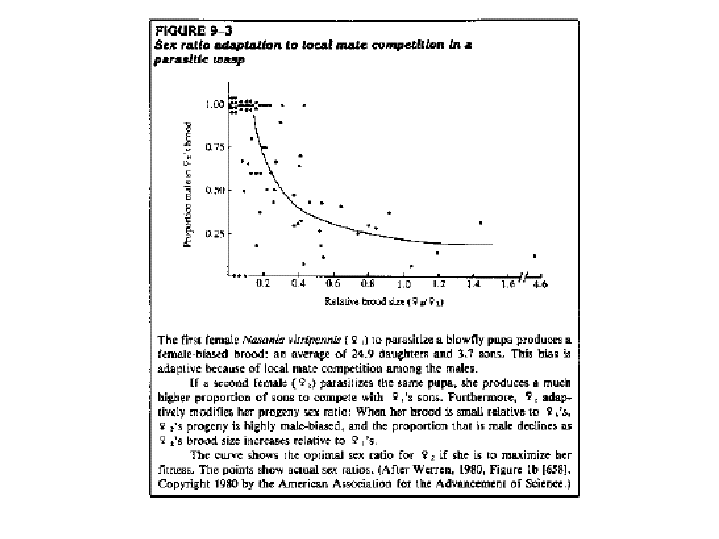
What happens when there are two (or more) foundresses (maternal females) placing eggs in a host?
One possibility is that they revert to a Fisherian sex ratio.
Another possibility is that they maintain a somewhat female biased sex ratio.
---
---
---
---
Can Hamiltonians win against Fisherians?
If Hamiltonian and Fisherian types of foundresses are equal frequency in the population then we can use a simplified binomial expansion to model the competition of these types.
If two females lay eggs in hosts then there will be groups in an 1:2:1 ratio
1 group of two H foundresses
2 groups of one H and one F foundresses
1 group of two F foundresses
we can then compare their overall fecundity
---
Two Hamiltonians producing a sex ratio of 3 to 17
3/6 * 34 +17 = 34 offspring for each female
Two Fisherians producing a ratio of 10 to 10
5/10 * 20 + 10 = 20 offspring for each female
One each in two groups
H 3/13 * 27 + 17 = 23.23; F 10/13 * 27 + 10 = 30.8
Hamiltonian total: 34 + 34 + 2*(23.23) = 114.5
Fisherian total: 20 + 20 + 2*(30.77) = 101.5 Hamiltonians win because of their high reproduction in "pure Hamiltonian" groups.
---
---
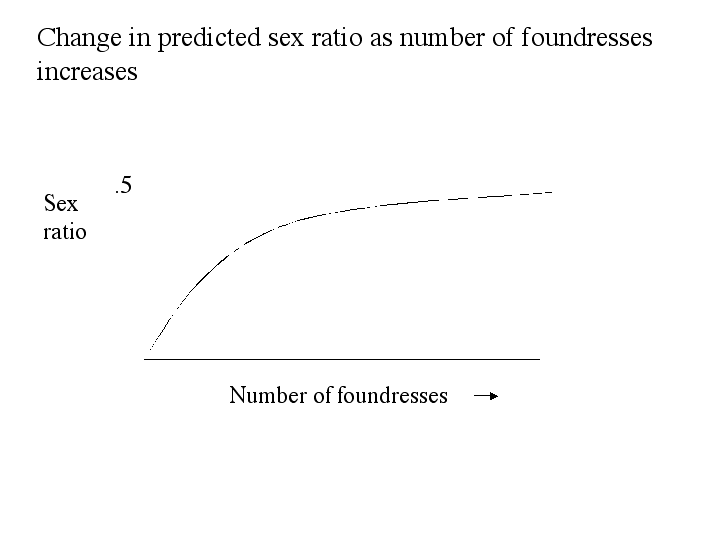
---
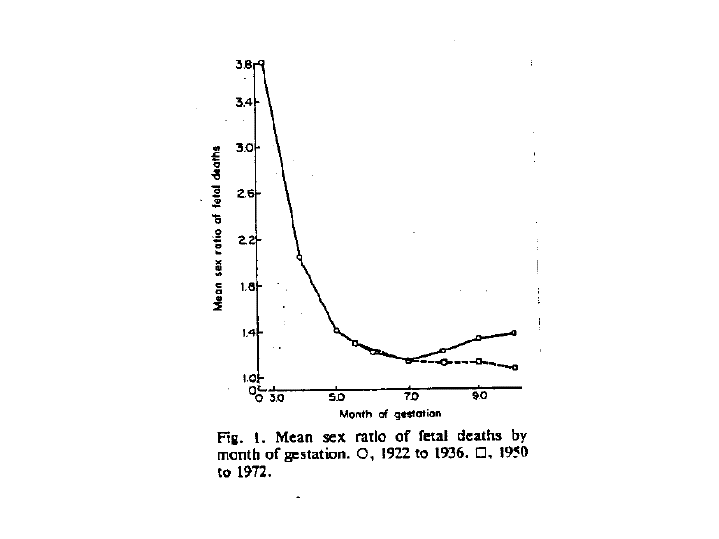
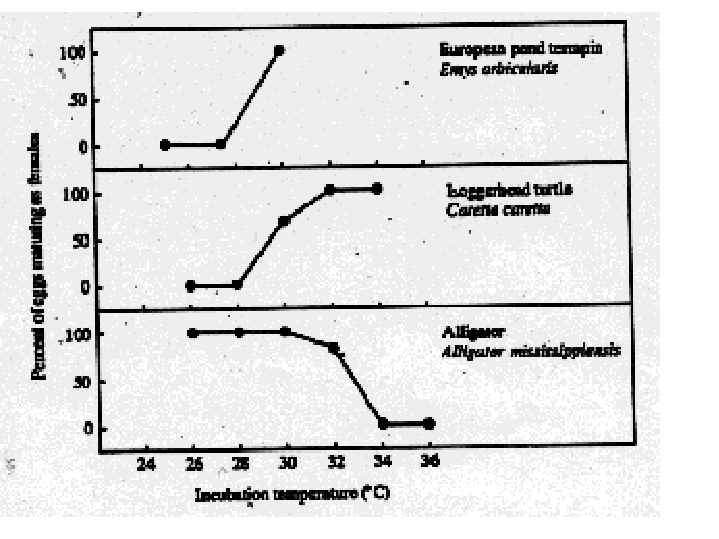
Environmentally Determined Sex Ratios
best know are in reptiles where temperature during fetal development determines sex
there is no one clear pattern
there are no sex chromosomes
---
---
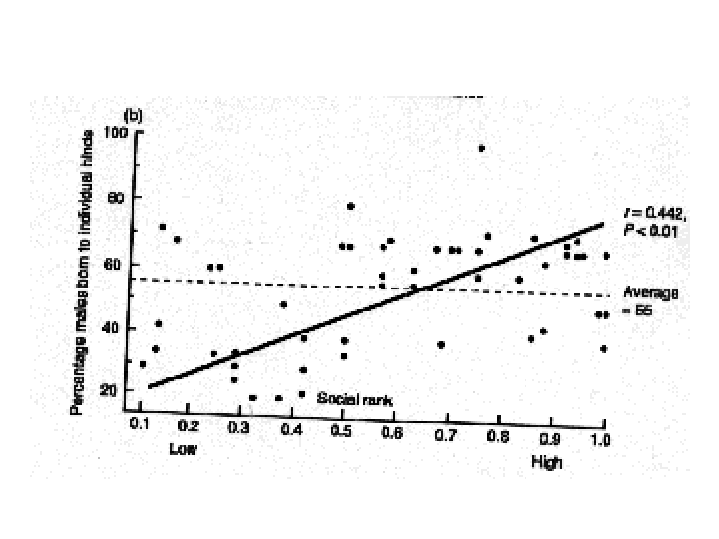
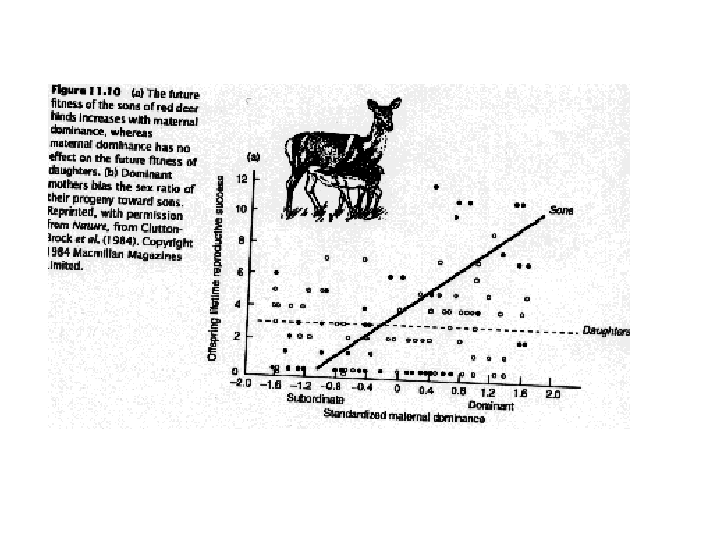
Trivers and Willard Hypothesis
In polygynous species high quality males have enhanced opportunity for reproduction
Females more likely to make high quality males should specialize in making males
---
---